The New Sudoku Players' Forum

[creint]

With my solver:
Only one step with one or more locked singles required.
Still easy.

[urhegyi]

twodoku1.gif (18.01 KiB) Viewed 1023 times

I think I finally found a more difficult variant I think it needs more advanced techniques. Can someone confirm this and pointing me in the right direction?
grid1

Code: Select all

.......4...92..7.3.3......6.1.76..5..75.......9.153.........32............3.47...

grid2

Code: Select all

...32.9............47.........683.4.......18..6..92.5.6......1.1.2..63...7.......

I think this has multiple solutions with r1c1 = 5 in 2nd grid (1sol) and with r1c1 = 8 in 2nd grid(3sol)

[creint]

It has multiple solutions, you can use more advanced techniques but with that you cannot reach beyond the following:

Code: Select all

7    28   1    6    3    9    28   4    5
45   6    9    2    8    45   7    1    3
2458 3    24   45   7    1    28   9    6
3    1    8    7    6    2    4    5    9
6    7    5    48   9    48   1    3    2
24   9    24   1    5    3    6    8    7
9    45   7    58   1    6    3    2    48   9    7    45
1    45   6    3    2    58   9    7    48   45   6    1
28   28   3    9    4    7    5    6    1    8    3    2
               7    5    1    6    8    3    2    4    9
               2    3    9    7    4    5    1    8    6
               48   6    48   1    9    2    7    5    3
               6    9    45   2    3    7    45   1    8
               1    8    2    4    5    6    3    9    7
               45   7    3    8    1    9    6    2    45

[urhegyi]

39721_350819.png (18.23 KiB) Viewed 968 times

Found an overlapping duosudoku I never saw before. The first time solving it I struggled a lot, but the 2nd time it was really nice to solve because I discovered a rule.
How would I best start when solving more sudokus like these?
This must be the solution when I have not made a mistake:
grid1:

Hidden Text: Show

Code: Select all

679431285481572693532698471294753168768124539153986724847219356315867942926345817

grid2:

Hidden Text: Show

Code: Select all

815726934326984715947531682681245397539867241472193568158679423263458179794312856

[urhegyi]

39394_290909.png (18.53 KiB) Viewed 964 times

Looking a second time the site sudoku.today had another example. So I know what to do tomorrow evening.
Was really easy now I understand the hidden constraints.
solution:
grid1:

Hidden Text: Show

Code: Select all

531289746297146853846537219428315967619728534753694128182473695964852371375961482

grid2:

Hidden Text: Show

Code: Select all

971468532465372198283159674197285346536941287824736951648523719759614823312897465

[1to9only]

The solution for the first puzzle is correct.

Code: Select all

.............726.................16...8.2.5.9.5..8...4.4...9.....5.......263....7 ED=2.3/1.2/1.2
...726.................16...8.2.5.9.5..8...4.4...9.....5.......263....7.......... ED=1.5/1.2/1.2


The second puzzle is of similar difficulty level, puzzle rating:

Hidden Text: Show

Code: Select all

..........97....5..4..3.2.9..8....6..1.7.8..4....94................5...1..59...82 ED=2.0/1.2/1.2
97....5..4..3.2.9..8....6..1.7.8..4....94................5...1..59...82.......... ED=1.5/1.0/1.0


[urhegyi]

Overlapping Sudoku (Daily Sudoku League #118).PNG (11.3 KiB) Viewed 943 times

I like the overlapping sudokus like the last two one, but they are hard to find on the internet and mostly very easy to solve. I'm searching for examples with naked and or hidden subsets, and also with x-wing. Can someone explain how they are created? Finally I found another one and give it a try.
solution:
grid1:

Hidden Text: Show

Code: Select all

936841257215937846847256931768312495423579618591684372384725169652193784179468523

grid2:

Hidden Text: Show

Code: Select all

159378462472569318683124957235796184916843725847251693521937846794685231368412579

[1to9only]

Code: Select all

......2....5..784..4..5...1...3.........7...8.9...4...3.....1...5...3..4..946..2. ED=1.5/1.2/1.2
.5..784..4..5...1...3.....7...7...8.9...4.........1...5...3..4..946..2....8...... ED=1.2/1.0/1.0


Can someone explain how they are created?

The process will be the same as discussed in: http://forum.enjoysudoku.com/how-to-create-a-sudoku-starting-from-a-solution-t38385.html.

- you'll need a solver for the overlapped puzzle.
- generate solution grids

- remove symmetric clues
- check solvable (unique solution)
- loop

- repeat until you have a puzzle of required difficulty
.

[creint]

If you don't care about that it must be minimal then you can rate ever time you remove a digit. It limits maximum difficulty but does not guarantee that difficulty.

In my solver within 10 seconds template input and a puzzle.
Here one with SE ~9, but not symmetric.

Code: Select all

. . . . . . . . . 
. . . . . . 9 4 . .
. 5 . . . 3 . 1 . .
. . . . . . . . . .
. . . . 8 . . . 3 .
. . . 4 . . 2 7 . .
. . . . . . . . . 2
1 . . . . 8 . 6 . .
. . 6 . . . 3 . . .
  9 . . . . . . 7 .

There are other ways for generating but this is the fastest way for small puzzles.

[1to9only]

I like the overlapping sudokus like the last two one, but they are hard to find on the internet and mostly very easy to solve. I'm searching for examples with naked and or hidden subsets, and also with x-wing.

Try these:
http://forum.enjoysudoku.com/wc2018-knockout-flowers-t34870.html
http://forum.enjoysudoku.com/wc2018-kazaguruma-special-t34829.html

Depends on what you are looking for: number of overlapped sudokus (2, 3, 5 (samurai), ... 105!), size of overlapped region (8x8 in the last one you posted), usually the overlapped region is 1 nonet (3x3 block) as in samurai, but can be 2-4 nonets for gattais with 2-4 sudokus. Some time back I posted a number of gattais in the Sudoku variants section, most/all are in the easy/medium difficulty level. I collected all the ones I posted and a few more, and stored them here: https://github.com/1to9only/Gattai-Puzzles.

For more gattais: Ruud's Specialty Puzzles at: http://www.rcbroughton.co.uk/sudoku/forum/viewtopic.php?f=4&t=13
.

[urhegyi]

If you don't care about that it must be minimal then you can rate ever time you remove a digit. It limits maximum difficulty but does not guarantee that difficulty.

In my solver within 10 seconds template input and a puzzle.
Here one with SE ~9, but not symmetric.

Code: Select all

. . . . . . . . . 
. . . . . . 9 4 . .
. 5 . . . 3 . 1 . .
. . . . . . . . . .
. . . . 8 . . . 3 .
. . . 4 . . 2 7 . .
. . . . . . . . . 2
1 . . . . 8 . 6 . .
. . 6 . . . 3 . . .
  9 . . . . . . 7 .

Is it really a SE9.0?
Solution:
grid1:

Hidden Text: Show

Code: Select all

491826537368751942752943816514372689627189453839465271285637194143298765976514328

grid2:

Hidden Text: Show

Code: Select all

687519423529438167143726895271894536394652718856371942432987651765143289918265374

There are other ways for generating but this is the fastest way for small puzzles.

[urhegyi]

In all 4 examples of the 8x8 overlapping grid I found this constraints:

grid1 r1c1 = grid2 r9c9
grid1 ri+1c1 = grid2 ric9

grid1 r1c1 = grid2 r9c9
grid1 r1ci+1 = grid2 r9ci

[Hajime]

In all 4 examples of the 8x8 overlapping grid I found this constraints: ....

Because there is an overlapping of 8 cells the outermost 2 cells (one per grid) must be equal.
But I confirm that the puzzle of creint is indeed very hard, so did you have a lucky guess or do you have extreme skills?

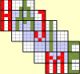
Here is a duo puzzle with 7x7 overlap:

duo_easy.png (5.37 KiB) Viewed 875 times

Solution is easy, only naked/hidden pairs,triples or quads needed.
In code:

Code: Select all

#2//B4/D8
....7..9......4....3....4.....................25.....6.....7.1.47.............2..
....4...........7..........5.....6.1...7.1.9..............2.......1....6..3......


And here a more difficult one. Same layout but chains are needed.

Code: Select all

#2//B4/D8
.....1..........67............4...............2.7...4..5....61.47.6......1.9...7.
.......3..4..............6..7...4.......61....6........9...7......1...5.........9


Because of the 7x7 overlap the 2 outermost pairs per row/col must be equal, but may be exchanged.

All generated with SiSeSuSo.exe

[1to9only]

The first 2 8x8 overlapped sudokus solve as JSBs (Jigsaw Sudoku with Block constraints).

Code: Select all

.............726.................16...8.2.5.9.5..8...4.4...9.....5.......263....7 ED=3.0
..........97....5..4..3.2.9..8....6..1.7.8..4....94................5...1..59...82 ED=1.2


So I generated some JSBs, and some can be solved as 8x8 overlapped sudokus!

Easy:

Code: Select all

. . . . . . . . .
. . 7 . 9 . . . . .
. . . . 2 6 5 . . .
. 2 8 . . . . 9 . .
. . . . . . 6 . . .
. . . 5 . 9 . . 7 .
. 3 . . . 7 . 8 . .
. 8 . . . . . . . .
. . . . . . . . 1 .
  . . . . . . . . .

...........7.9........265...28....9.......6.....5.9..7.3...7.8..8...............1 ED=1.5/1.2/1.2
.7.9........265...28....9.......6.....5.9..7.3...7.8..8...............1.......... ED=2.0/1.2/1.2


Medium:

Code: Select all

. . . . . . . . .
. . . . 7 . 9 . . .
. 8 . . . . . 3 . .
. 7 . . . . 2 . . .
. . 5 4 6 . . . 8 .
. 6 . . . . 3 . . .
. . . . . . . 5 . .
. . . 1 . . . . 4 .
. . 4 . 5 . . . . .
  . . . . . . . . .

.............7.9...8.....3..7....2....546...8.6....3.........5....1....4..4.5.... ED=2.6/1.2/1.2
...7.9...8.....3..7....2....546...8.6....3.........5....1....4..4.5.............. ED=4.1/1.2/1.2


Hard:

Code: Select all

. . . . . . . . .
. 7 . . . . 1 . . .
. . . . 3 . . . 8 .
. 6 . . . . 9 2 . .
. . 9 . 8 5 . . . .
. 5 . . . 1 . . . .
. . . 4 . 7 . . 3 .
. 2 . . . . . . . .
. . . . . . 7 . . .
  . . . . . . . . .

..........7....1......3...8.6....92...9.85....5...1......4.7..3.2.............7.. ED=7.2/1.2/1.2
7....1......3...8.6....92...9.85....5...1......4.7..3.2.............7............ ED=6.6/1.2/1.2


[Hajime]

The first 2 8x8 overlapped sudokus solve as JSBs (Jigsaw Sudoku with Block constraints).

Code: Select all

.............726.................16...8.2.5.9.5..8...4.4...9.....5.......263....7 ED=3.0
..........97....5..4..3.2.9..8....6..1.7.8..4....94................5...1..59...82 ED=1.2


Is this code correct?

So I generated some JSBs, and some can be solved as 8x8 overlapped sudokus!

Easy: Singles only confirmed
Medium: Naked/Hidden Pairs/Triplets and Pointing/Claiming is confirmed
Hard: Besides Basic Methods I needed an X-chain and an XY-chain (bivalue) both of length 6 (and some Skyscrapers).