The New Sudoku Players' Forum

[denis_berthier]

.
Do you have similar black holes for vanilla Sudoku? Does it apply anything on their complexity?
.

[m_b_metcalf]

.
Do you have similar black holes for vanilla Sudoku? Does it apply anything on their complexity?
.

By vanilla I assume you mean based on non-X 16x16 grids. I'm not sure it's possible for such a puzzle to have a completely empty central hole. It would have to be constructed from a 16x16 grid which yields a single solution when each 8x8 corner, in turn, is removed. I haven't found a grid where any corner can be removed.

Here's a puzzle where the centre is nearly as empty as possible, and with rotational symmetry on the outside. It takes my program 16s to solve.

Code: Select all

  . 12  . 11 14  .  .  .  .  3 15  .  . 13  .  7  .  9  . 10  .  .  .  8 16  .  1  .  6  .  . 14
  .  .  .  .  .  .  4  .  .  .  .  1  5  .  . 11  .  .  .  . 10  .  .  .  .  .  3  . 12  .  . 13
  .  .  .  . 11  .  .  6  2 16  .  7 12 14 15  .  .  4  .  .  1  .  .  .  .  9  . 13  8  .  . 10
  .  .  .  .  . 15  5 12  .  .  .  .  . 16  .  3  . 12 14  1  .  .  2  9  .  .  4 15  .  .  .  .
 15 11  .  .  .  .  .  .  .  . 16  8  .  .  9  .  .  .  .  .  4  .  .  . 10  8  .  5  2  .  . 16
  .  . 12  .  4  . 16  8  .  1  .  .  .  3  .  2  .  .  .  .  8  6 10  .  .  2  . 16  .  .  .  7
  2  6  .  7  .  .  .  .  .  4  . 10  1  .  .  .  .  2  3  .  7  .  . 11 12  .  .  1  9 10  .  6
  .  . 13  8  .  9  . 15 12  7  .  . 11  6  .  .  .  .  5  7  .  .  .  .  .  . 14  .  .  .  4 12
  7  .  .  9  .  .  6  .  . 11  .  .  8  .  .  . 16  .  .  .  3  .  .  .  .  .  . 14  .  .  .  .
  .  .  .  .  8  .  3  .  7  .  .  . 13  .  .  .  .  .  .  .  .  .  .  .  .  7 16  .  .  .  .  .
  .  . 10 12  .  .  .  2  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  . 15  .  . 16  3  .  1
 14  .  .  .  .  1  . 13  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  . 11  .  7  .  .  .
 11  .  . 15  .  . 12  1  .  .  .  .  .  .  .  .  .  .  .  .  .  1  .  .  .  .  2  4 10  8  9  .
  . 10  .  5  .  7 15  4  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  8  . 16  .  2
  8  .  . 16  .  5  .  .  .  .  .  . 10  .  .  .  .  .  .  .  .  . 11  . 15 13  .  .  3  7  .  .
  .  .  7  . 10  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  9  .  .  .  5  .  .  .  6  .
  . 16  .  .  .  3  .  .  .  .  8  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  . 15  .  4  .  .
  .  .  4  3  .  . 12 13  .  .  .  .  .  5  .  .  8  .  .  .  .  .  .  .  .  .  2  .  5  .  .  6
  5  .  1  . 11  .  .  .  .  .  .  9  .  .  .  .  .  .  .  .  .  .  . 11 12  6  4  .  3  . 16  .
  .  7 15 10  4  6  .  .  .  .  .  .  .  .  .  .  .  4  .  .  .  .  .  .  3 16  .  . 13  .  .  2
  .  .  .  4  . 14  .  .  .  .  .  5  .  .  .  .  .  .  .  .  .  6  .  .  2  .  1  .  .  .  .  7
  7  .  6  9  .  .  5  .  .  .  .  .  . 13 14  .  .  5  .  .  .  .  .  . 15  .  .  .  1 11  .  .
  .  .  .  .  .  1 13  .  .  .  .  .  .  .  . 10  .  . 13  .  .  .  .  .  . 12  .  7  .  .  .  .
  .  .  .  . 15  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  .  8  .  .  9  .  .  5
  1 11  .  .  .  2  .  .  .  .  .  . 15 10  .  .  .  . 10  6  .  . 13  4  1  .  9  .  7  8  .  .
  9  .  3  7 12  .  . 15 14  .  .  6  .  8  2  .  .  .  . 13 16  .  9  .  .  .  .  . 10  . 12 15
  2  .  .  .  1  .  4  .  . 13  7 15  .  .  .  .  9  .  2  .  .  .  8  . 11 15  . 16  . 14  .  .
 10  .  .  5  6  . 11  3  .  .  .  2  .  .  .  .  .  7  .  .  6  2  .  .  .  .  .  .  .  .  1 16
  .  .  .  . 13 10  .  .  1  9  .  . 14 11 16  . 10  .  9  .  .  .  .  .  5 11 16  .  .  .  .  .
 14  .  . 11  2  .  6  .  .  .  . 16  .  .  7  .  .  6  8  4  2  .  1 16  9  .  .  3  .  .  .  .
 15  .  .  6  .  8  .  .  .  .  . 14  .  .  .  . 13  .  .  5 14  .  .  .  .  4  .  .  .  .  .  .
 12  .  .  1  .  4  . 16 10  .  .  .  8  .  3  .  1  . 12  .  .  9  5  .  .  .  . 14  2  . 11  .

Does that help?

Regards,

Mike

[denis_berthier]

.
Indeed, I meant ordinary/standard Sudoku grids (9x9, 16x16, 25x25, ...)
.

[m_b_metcalf]

Indeed, I meant ordinary/standard Sudoku grids (9x9, 16x16, 25x25, ...)

Denis,
I'm not sure what your real question is. In any case, my definition of a 'Black Hole' puzzle, given in the first post of this thread, is valid only for even box size.

Regards,

Mike

[Hajime]

.
Do you have similar black holes for vanilla Sudoku? Does it apply anything on their complexity?
.

9x9 Sudoku's are odd numbered, also boxes of 3x3 are odd numbered.
If you place 4 Sudokus in a square the 5th Sudoku cannot include/exclude the middle cell r5c5 in all 4 others.
But you can tweak the positions of the 4 adjacent Sudokus, like this:

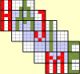
The center cell in the middle of the puzzle belongs to the 5th Sudoku only, not to the other 4. The overlapping 4 parts are all 4x5=20 cells.
And now one can try to make a puzzle with a black hole, by eliminating all givens in the 5th Sudoku.
I can make the example below with just 1 given, but not 0 givens.

Code: Select all

                                    +-----------+-----------+-----------+       
                                    |           | 7         |     3     |       
+-----------+-----------+-----------+           |           |           |       
| 2         | 6   5     | 1         |         1 |           | 9   5     |       
| 1         |     2     | 8         |           | 2   5     |           |       
|           |           |           +-----------+-----------+-----------+       
|           |     4     |           | 3         |           | 4         |       
+-----------+-----------+-----------+           |           |           |       
|           | 2   7     |         1 |           |     6     |           |       
|           |       +---+-------+---+-------+---+-------+   |           |       
|           | 4   6 |           |           |           |   |           |       
|           |       |           |           |           +---+-----------+       
|           |     3 |           |           |           |   |     9   7 |       
+-----------+-------+           |           |           |   |           |       
|     3   6 |       |           |           |           |   |         4 |       
|           |       +-----------+-----------+-----------+   |           |       
|     8     |       |           |           |           |   |           |       
|           |       |           |           |           +---+---+-------+---+   
|         5 |       |           |           |           | 5     |           |   
+---+-------+---+---+           |           |           |       |           |   
    |     6     |   |           |           |           |     3 |           |   
    |           |   +-----------+-----------+-----------+       |           |   
    |           |   |           |           |           |       |           |   
    |           |   |           |           |           +-------+-----------+   
    |           | 4 |           |           |           |       |     6     |   
    +-----------+---+           |           |           |       |           |   
    | 6         |   |         3 |           |           |       | 7       3 |   
    |           |   +-------+---+-------+---+-------+---+       |           |   
    | 9         |           | 5         |           |           |           |   
    |           |           |           +-----------+-----------+-----------+   
    |     1     | 7         |           |           |     3   6 |           |   
    +-----------+-----------+-----------+           |           |           |   
    |           |         6 |           |     6     |     1     |         9 |   
    |           |         2 | 7   9     | 5       4 |           |           |   
    |           |           |           +-----------+-----------+-----------+   
    |     4     | 5         |           |                                       
    +-----------+-----------+-----------+                                       

#5//B6/K4/C24/L22/G14
2..65.1..1...2.8......4.......27...1...46........3.....36.......8.........5......
...7...3...1...95....25....3.....4......6....................97........4.........
.6...................4.....6.....3..9.....5...1.7..........6........279..4.5.....
....5.........3...................6.......7.3.............36....6..1...95.4......
..........................................................................3......


Not all border lines are drawn.
Puzzle can be solved with basic methods: Pointing/Claiming and Hidden/Naked Pairs/Triplets/Quads

[m_b_metcalf]

Hajime,

Nice idea, but I'd rather call it a squashed samurai than a black hole. The solution's below, and my program claims the puzzle is minimal. The clue in the middle sub-puzzle is sitting on a UA4, that's why it can't be removed.

Regards,

Mike

Hidden Text: Show

Code: Select all

                   5 2 8 7 9 4 6 3 1     
 2 9 8 6 5 7 1 3 4 7 4 1 6 8 3 9 5 2     
 1 4 7 9 2 3 8 6 5 9 6 3 2 5 1 7 4 8     
 6 5 3 8 4 1 9 2 7 3 8 5 9 1 2 4 7 6     
 3 6 9 2 7 5 4 8 1 2 9 4 3 6 7 1 8 5     
 8 7 1 4 6 9 3 5 2 1 7 6 8 4 5 3 2 9     
 5 2 4 1 3 8 6 7 9 4 5 2 1 3 6 8 9 7     
 7 3 6 5 9 4 2 1 8 6 3 9 5 7 8 2 1 4     
 4 8 2 7 1 6 5 9 3 8 1 7 4 2 9 5 6 3     
 9 1 5 3 8 2 7 4 6 5 9 1 3 8 5 4 6 2 7   
   5 6 9 2 3 1 8 4 7 2 5 6 9 7 3 4 8 1   
   4 8 7 6 5 9 2 1 3 8 4 7 6 2 1 3 9 5   
   1 2 3 4 7 8 6 5 9 4 3 2 1 9 7 5 6 8   
   6 5 8 9 1 4 3 7 2 6 8 9 5 4 2 7 1 3   
   9 7 4 8 2 3 5 6 1 1 7 5 3 6 8 9 4 2   
   3 1 2 7 6 5 9 8 4 7 9 1 2 3 6 8 5 4   
   7 9 1 3 8 6 4 2 5 3 6 8 4 1 5 2 7 9   
   8 3 5 1 4 2 7 9 6 5 2 4 7 8 9 1 3 6   
   2 4 6 5 9 7 1 3 8                     

[creint]

Whole puzzle not minimal.
Only requires 2 layers of locked singles (pointing/claiming).

Here is one minimal SE < 10.

Hidden Text: Show

Code: Select all

                  . . . 7 . . . 3 .
2 . . 6 5 . 1 . . . . 1 . . . 9 5 .
1 . . . . . 8 . . . . . 2 5 . . . .
. . . . 4 . . . . 3 . . . . . 4 . .
. . . . 7 . . . . . . . . 6 . . . .
. . . 4 . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . 9 7
. 3 . . . . . . . . . . . . . . . 4
. 8 . . . . . . . . . . . . . . . .
. . 5 . . . . . . . . . . . 5 . . . .
  . 6 . . . . . . . . . . . . 3 . . .
  . . . . . . . . . . . . . . . . . .
  . . . 4 . . . . . . . . . . . . 6 .
  6 . . . . . 3 . . . . . . . . 7 . 3
  9 . . . . . 5 . . . . . . . . . . .
  . 1 . 7 . . . . . . . . . 3 6 . . .
  . . . . . 6 . . . . 6 . . 1 . . . 9
  . . . . . 2 7 9 . 5 . 4 . . . . . .
  . 4 . 5 . . . . .

[blue]

Here's one with an 11x11 hole and empty edges.

Code: Select all

                  . . . . . . . . . 
. . . . . . . . . 7 . 6 . . . . 9 . 
. 8 . 9 . . . . . . 4 9 . 8 . . 5 . 
. . . . 3 . . 4 . 3 . . . 2 1 . 4 . 
. . . . . . . . . . . . . . . 6 . . 
. . 1 8 . . . . . . . . . . . . . . 
. . 5 . . . . . . . . . . . . 8 . . 
. 1 7 . . . . . . . . . . . . . . . 
. . . . . . . . . . . . . . . . . . 
. 3 8 . . . . . . . . . . . . . 5 3 .
  . . . . . . . . . . . . . . . 4 . .
  . . 8 . . . . . . . . . . . . 6 . .
  . . 9 . . . . . . . . . . . . 8 . .
  . . . . . . . . . . . . . . . . . .
  . 1 5 . . . . . . . . . . . 2 . . .
  . 6 . 7 3 . . . . . 1 5 . 8 . . . .
  . . . . 6 9 . 2 . . . . . 1 3 . 7 .
  . 9 . . . . . . 1 . . . . . . . . .
  . . . . . . . . .                 

..........8.9.........3..4............18.......5.......17................38......
.........7.6....9..49.8..5.3...21.4.......6.................8....................
...........8........9................15.......6.73........69.2..9......1.........
......53.......4........6........8................2....15.8........13.7..........
.................................................................................

(I don't have the tools to rate it).

[creint]

Here's one with an 11x11 hole and empty edges.
(I don't have the tools to rate it).

SE < 10 in 288 seconds
Is minimal 4 seconds.

[urhegyi]

Needed 2 guesses:

[Hajime]

Nice idea, but I'd rather call it a squashed samurai than a black hole.

I agree, my first idea is also: it is an ugly duck.

Here's one with an 11x11 hole and empty edges.

What a beauty. My ugly duck is transformed into an elegant swan.

Needed 2 guesses:

How did you do that? Sisesuso needs a lot more

[urhegyi]

I have created another one with Hajime's style requirements:

Code: Select all

#5//B6/K4/C24/L22/G14
6.....8.....92......96...4.8............4..............1.2......4................
.......86.....5.....9.....73...2.....2..............29.....2...................7.
5.1......4..........9.................5.....3...7.....1.......4..64......8.......
.......................1........4.1.......2.6..1....4.7...8.........3.7...9.....8
.................................................................................


[blue]

I have created another one with Hajime's style requirements

Code: Select all

                  . . . . . . . 8 6
6 . . . . . 8 . . . . . . . 5 . . .
. . . 9 2 . . . . . . 9 . . . . . 7
. . 9 6 . . . 4 . 3 . . . 2 . . . .
8 . . . . . . . . . 2 . . . . . . .
. . . . 4 . . . . . . . . . . . 2 9
. . . . . . . . . . . . . . 2 . . .
. 1 . 2 . . . . . . . . . . . . . .
. 4 . . . . . . . . . . . . . . 7 .
. . . . . . . . . . . . . . . . . . .
  5 . 1 . . . . . . . . . . . . . . .
  4 . . . . . . . . . . . . . 1 . . .
  . . 9 . . . . . . . . . . . 4 . 1 .
  . . . . . . . . . . . . . . . 2 . 6
  . . 5 . . . . . 3 . . 1 . . . . 4 .
  . . . 7 . . . . . 7 . . . 8 . . . .
  1 . . . . . . . 4 . . . . . 3 . 7 .
  . . 6 4 . . . . . . . 9 . . . . . 8
  . 8 . . . . . . .

Validated: minimal puzzle with unique solution.

Hidden Text: Show

Code: Select all

                  5 1 3 7 9 4 2 8 6
6 2 3 4 5 1 8 7 9 7 8 6 2 1 5 3 9 4
1 8 4 9 2 7 3 5 6 2 4 9 3 8 6 1 5 7
7 5 9 6 3 8 2 4 1 3 9 5 6 2 1 7 4 8
8 7 2 5 1 9 4 6 3 8 2 7 4 5 9 6 1 3
3 6 1 8 4 2 7 9 5 4 6 1 8 3 7 5 2 9
4 9 5 3 7 6 1 8 2 9 3 4 5 7 2 8 6 1
9 1 7 2 6 4 5 3 8 1 7 2 9 6 8 4 3 5
5 4 6 1 8 3 9 2 7 6 5 8 1 4 3 9 7 2
2 3 8 7 9 5 6 1 4 3 8 9 7 2 4 6 5 3 1
  5 3 1 6 7 8 4 9 2 1 6 3 5 9 7 4 8 2
  4 2 8 5 9 3 6 1 7 4 5 2 8 3 1 6 9 7
  6 7 9 2 1 4 5 3 8 2 7 6 9 5 4 8 1 3
  9 4 3 1 8 2 7 6 5 9 3 4 1 7 8 2 5 6
  7 1 5 9 4 6 2 8 3 5 8 1 3 6 2 7 4 9
  8 6 2 7 3 5 1 4 9 7 1 5 6 8 9 3 2 4
  1 5 7 8 6 9 3 2 4 6 2 8 4 1 3 9 7 5
  3 9 6 4 2 7 8 5 1 3 4 9 7 2 5 1 6 8
  2 8 4 3 5 1 9 7 6


[blue]

Black holes ...

A 13x13 hole, with empty edges.

Code: Select all

                  . . . . . . . . . 
. . . . 7 1 5 . . . 8 9 . . . 3 . . 
. . . 9 . . 3 . 1 . 6 . 2 . . 5 . 1 
. 4 9 . . . . . . . . . . . . . 6 . 
. 9 . . . . . . . . . . . . . . . 4 
. . . . . . . . . . . . . . . . . 9 
. . 6 . . . . . . . . . . . . . 3 7 
. 3 . . . . . . . . . . . . . . . . 
. 1 8 . . . . . . . . . . . . . . . 
. . . . . . . . . . . . . . . . . . .
  . . . . . . . . . . . . . . . 3 2 .
  . . . . . . . . . . . . . . . . 5 .
  7 5 . . . . . . . . . . . . . . 8 .
  2 . . . . . . . . . . . . . . . . .
  5 . . . . . . . . . . . . . . . 1 .
  . 4 . . . . . . . . . . . . . 1 9 .
  6 . 3 . . 2 . 9 . 5 . 1 . . 8 . . .
  . . . 1 . 7 . 2 . . . 7 . 3 . 4 . .
  . . . . . . . . .                 

....715.....9..3.1.49.......9..................6.......3........18...............
..........89...3...6.2..5.1.......6.........4........9.......37..................
..................75.......2........5.........4.......6.3..2.9....1.7.2..........
...............32........5........8.................1.......19.5.1..8.....7.3.4..
.................................................................................

A 15x15 hole.

Code: Select all

                  . . . 3 . 6 9 . . 
. . 7 . 2 5 . 8 1 9 . . 1 . . . 4 . 
. 3 . . . . . . . . . . . . . . . 6 
6 . . . . . . . . . . . . . . . . 5 
2 5 . . . . . . . . . . . . . . . . 
. 6 . . . . . . . . . . . . . . . 9 
4 . . . . . . . . . . . . . . . . 7 
. 2 . . . . . . . . . . . . . . . . 
8 . . . . . . . . . . . . . . . . 3 
. . . . . . . . . . . . . . . . . . .
  8 . . . . . . . . . . . . . . . . 2
  . . . . . . . . . . . . . . . . 1 .
  1 . . . . . . . . . . . . . . . 4 9
  3 . . . . . . . . . . . . . . . . .
  . . . . . . . . . . . . . . . . 6 5
  4 . . . . . . . . . . . . . . . . 6
  9 . . . . . . . . . . . . . . . 8 .
  . 3 . . . 1 . . 7 3 4 . 5 7 . . . .
  . . 6 2 . 5 . . .                 

..7.25.81.3.......6........25........6.......4.........2.......8.................
...3.69..9..1...4.........6........5.................9........7.................3
8.................1........3.................4........9.........3...1..7..62.5...
.................2.......1........49................65........6.......8.34.57....
.................................................................................

[blue]

Last one: a round hole with radius ~8.8

Code: Select all

                  . 4 1 . 6 . 8 . .
. . . 6 1 2 . . . . . . . 3 7 1 . .
. . . 8 . . . . . . . . . . . 7 5 .
8 4 7 . . . . . . . . . . . . . 7 9
7 9 . . . . . . . . . . . . . . . 5
4 1 . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . .
1 . . . . . . . . . . . . . . . . .
5 . . . . . . . . . . . . . . . . .
. . . . . . . . . . . . . . . . . . .
  . . . . . . . . . . . . . . . . . 7
  . . . . . . . . . . . . . . . . . 6
  . . . . . . . . . . . . . . . . . .
  . . . . . . . . . . . . . . . . 2 8
  6 . . . . . . . . . . . . . . . 3 9
  1 5 . . . . . . . . . . . . . 5 . 2
  . 1 7 . . . . . . . . . . . 3 . 7 .
  . 8 3 2 . . . . . . . . 5 1 4 . . .
  . . 5 . 3 . 2 4 .

...612......8.....847......79.......41................1........5.................
.41.6.8......371........75........79........5....................................
....................................6........15........17.......832.......5.3.24.
.................7........6................28.......39......5.2.....3.7....514...
.................................................................................