The New Sudoku Players' Forum

[StrmCkr]

this puzzle construct isn't my idea a user on my sudoku reddit page posted

https://logic-masters.de/Raetselportal/Raetsel/zeigen.php?id=000HSF

this is a neat construct:

a nxn puzzle with n+j no-net cages where the the sum of the cage is a Prime number

each row & col can only have the n digits listed once.

enjoy

[HATMAN]

Well found StrmCkr
Really neat - that is the definition of minimal!

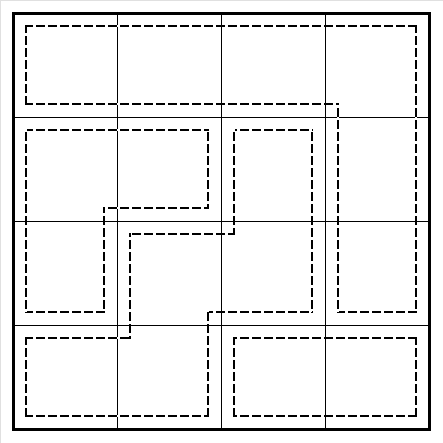
I am going to do a few climbing the sizes here is 4:

Prime Killer 4
removed not unique
Luckily the solution I missed is maximum repeat - so reinstated.
Standard Sudoku rules: every row and column contains the digits from 1 to 4 exactly once each.
Prime cages: the digits in each cage sum to a distinct prime number. The digits in a cage must repeat the maximum possible number of times (if it is possible to do so), but the prime sums do not repeat.

[HATMAN]

Prime Killer 6

Standard Sudoku rules: every row and column contains the digits from 1 to 6 exactly once each.
Prime cages: the digits in each cage sum to a distinct prime number. The digits in a cage may repeat, but the prime sums do not repeat.

I think it is unique without the 17 clue, but too much alternative eliminiation for my taste.