The New Sudoku Players' Forum

[Mathimagics]

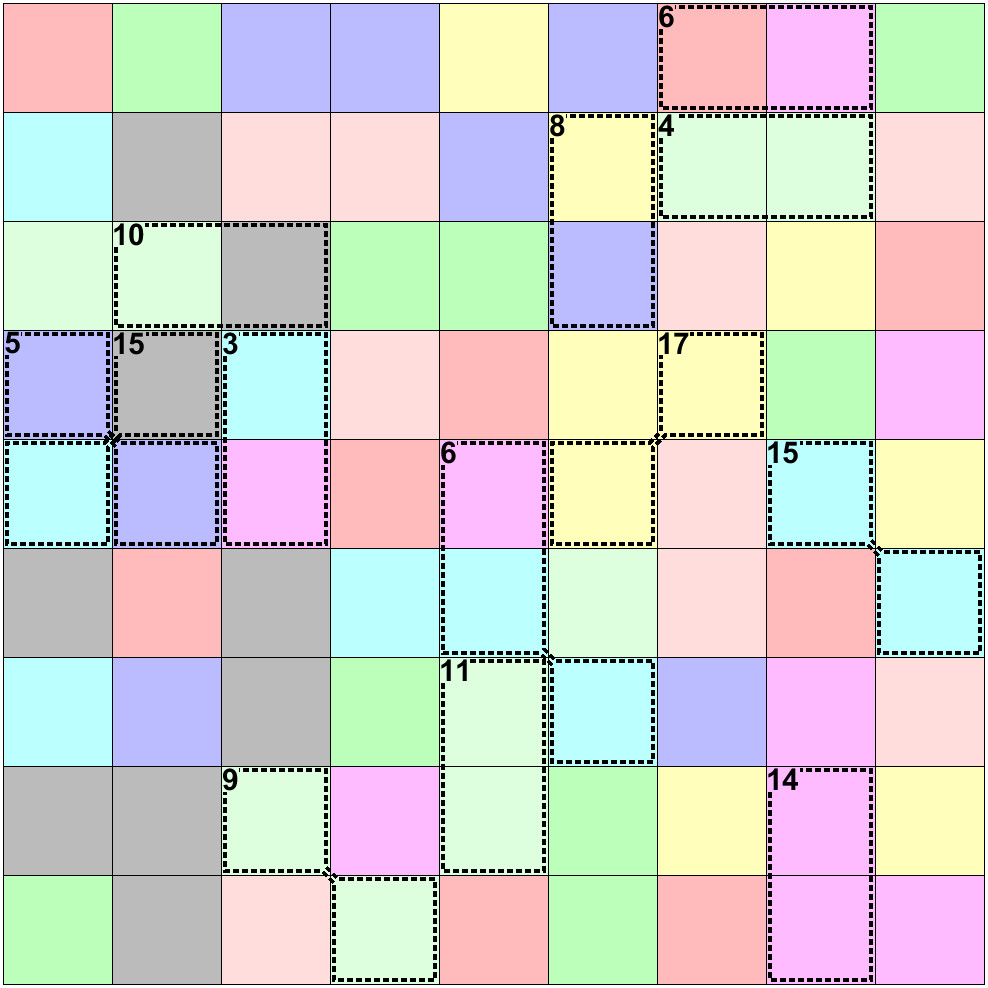
A couple of examples of Sudoku Jigsaws (with non-contiguous regions) which require just 8 clues.

Jigsaw-8clue-Examples.gif (14.95 KiB) Viewed 1287 times

If one considers the set of possible Jigsaw patterns, there are obviously far more than there are Latin Squares.

For N=9, we have 377,597,570,964,258,816 different Latin Squares, which is quite a lot, but tiny by comparison with the number of possible Jigsaw patterns, which is a whopping

146,413,934,927,214,422,927,834,111,686,633,731,590,253,260,933,067,148,964,500,000,000.

Now this includes all possible partitions of the 81 cells into 9 regions of equal size, with no restriction on whether these regions are joined together or not.

I generated over 1,000 random examples (admittedly a drop in the ocean), and found that in EVERY case, an 8-clue Jigsaw puzzle was possible.

When we consider only "conventional" Sudoku Jigsaw's, ie with 9 contiguous regions, that's when the average number of clues required tends to increase, with the standard Sudoku puzzle (17-clue minimum) representing a "worst case" from the minimum-clue perspective (although we know there exist 8-clue jigsaws with contig regions, these are exceptional cases)

[HATMAN]

Thank you Jim, 1 is enlightened.


Here is a zero killer based on your left hand one with the same solution. It's very hard - a lot of fishes.



This one is more reasonable.

[tarek]

As you found, when your jigsaw regions skew away from the vanilla sudoku format, the grids become more RESTRAINED. This is also true when you add extra regions like Windoku for instance.

I have (and so did Hatman) posted examples of restrained sudoku variants with 8 clues only in the distant past!!!

http://www.rcbroughton.co.uk/sudoku/forum/viewtopic.php?f=13&t=851&view=next

Tarek

[Mathimagics]

Yes, I believe that the size of the minimum critical set (ie minimum clue set) increases as the number of traversals in the Jigsaw template increases.

The actual number for a given puzzle will also depend on the structure of the LS that is the solutuon.

Regular Sudoku (indeed any rectangular region format) has the maximum number of traversals (46,656). At the other end of the spectrum a Jigsaw template that is itself a Latin Square might only have a couple of hundred traversals (250 - 350).