The New Sudoku Players' Forum

[re'born]

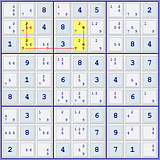
That one has already been classified by Mike Barker as an UR+2kd. See here. Very nice example of the technique in action.

RW

Ah, so it has. I'm not sure how I missed that one all this time. Thanks, RW.

[Luke]

These UR's with multiple extras are intriguing, but I don't really get it!

Code: Select all

 *--------------------------------------------------------------------*
 | 56     26     462    | 1458   7      9      | 3      4612   1248   |
 | 1      9      4627   | 45+8   3      48     | 45+278 4627   248    |
 | 357    37     8      | 45+1   2      6      | 45+7   #147    9      |
 |--------------------------------------------------------------------|
 | 4      2378   1      | 78     5      2378   | 289    239    6      |
 | 368    5      9      | 468    1468   12348  | 248    234    7      |
 | 3678   23678  2367   | 46789  4689   23478  | 1      5      2348   |
 |----------------------+----------------------+----------------------|
 | 2      178    5      | 3      1489   1478   | 6      1479   14     |
 | 36789  13678  367    | 2      14689  1478   | 479    13479  5      |
 | 3679   4      367    | 679    169    5      | 279    8      123    |
 *--------------------------------------------------------------------*

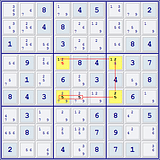
...since its going crazy anyway: what about the elimination rep'nA found in the grid MJ showed above? You have 2 strong links for the 5 and a "finned" strong link for the 4 to eliminate 4 in r2c7.

Hmmm. It is pretty easy to show that when you have just a "strong elbow" in a generic UR(+4, though it doesn't have to be +4)...

Code: Select all

abX-----abZ
     a  /|
         |
     /  a|
         |
  /      |
abY     abW

...the the 'b' candidate in the corner of the elbow is weakly linked to either the 'a' or 'b' candidates in the cell in the opposite corner. In other words, if either 'a' or 'b' are true in the abY-cell then b is false in the abZ-cell, and vice-versa. Any conjugate or strong link between the 'b' in the abY-cell and any grouping of 'b's sharing a house with the abZ-cell will thus kill the 'b' in the abZ-cell. This particular case is in the realm of an AUR, but it is quite obvious to see--probably as easy to spot as a type 3/UR assisted locked set deduction.

Graphically, it looks like this.



The 5's meet in a "strong elbow" and the other base candidate in the elbow is eliminated: the red 4. The 4 candidate is involved in no strong links in the rectangle.

So here's another puzzle with the same setup, as far as I can tell.
It's a Daily Nightmare, Dec 12, 07. There's one "strong elbow," and no other strong link on either base candidate.



Problem is, the correct value for r9c6 is 9, which I had thought would be the elimination. The answer must be that this puzzle has no "finned strong link" on 9, but that's where I'm getting lost....

Luke in Ca



Thanks to The Daily Nightmare, 12 14 2007:

Code: Select all

 *-----------*
 |..5|...|83.|
 |.3.|7.1|..9|
 |...|.6.|...|
 |---+---+---|
 |...|...|36.|
 |1..|.4.|..2|
 |.56|...|...|
 |---+---+---|
 |...|.3.|...|
 |2..|6.4|.9.|
 |.84|...|7..|
 *-----------*


 
 
 *--------------------------------------------------------------------*
 | 67     167    5      | 4      29     29     | 8      3      17     |
 | 48     3      28     | 7      5      1      | 6      24     9      |
 | 79     24     179    | 38     6      38     | 1245   12457  1457   |
 |----------------------+----------------------+----------------------|
 | 4789   24     2789   | 1589   1789   5789   | 3      6      14578  |
 | 1      79     3789   | 3589   4      6      | 59     578    2      |
 | 34789  5      6      | 12389  12789  23789  | 149    1478   1478   |
 |----------------------+----------------------+----------------------|
 | 5      1679   179    | 128    3      278    | 124    1248   1468   |
 | 2      17     137    | 6      178    4      | 15     9      1358   |
 | 36     8      4      | 1259   129    259    | 7      12     36     |
 *--------------------------------------------------------------------*


[ronk]

Luke451, you are missing ravel's and Myth's usage of a 3rd strong link.

You have 2 strong links for the 5 and a "finned" strong link for the 4 to eliminate 4 in r2c7.

Any conjugate or strong link between the 'b' in the abY-cell and any grouping of 'b's sharing a house with the abZ-cell will thus kill the 'b' in the abZ-cell.

In order to avoid a "deadly pattern", at least one of the extra candidates in r23c47 must be true. In AIC notation, the strong inference set is (278)r2c7 = (8)r2c4 = (7)r3c7 = (1)r3c4.

Code: Select all

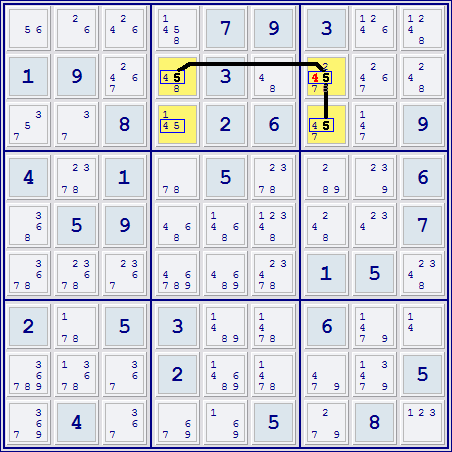
 56    26    246   | 1458  7     9     | 3      1246  1248
 1     9     2467  | 45+8  3     48    | 45+278 2467  248
 357   37    8     | 45+1  2     6     | 45+7   147   9

Code: Select all

(278)r2c7
  ||
(8-5)r2c4 = (5)r2c7
  ||
(7-5)r3c7 = (5)r2c7
  ||
(1-4)r3c4 = (4)r3c78 ==> r2c7<>4

[Luke]

Wow. I'm feelin' kinda lame right now..

I wish I could say I understood one single part of your response. I know what AIC's are and I usually can follow the strong-weak path in AIC notation....but I've never seen the likes of the notation you're using.

278=8=7=1. Okay, those are the extra candidates. At least one must be true. I got it that far. I guess there's some way to run an AIC chain on these extra candidates?? Where does the "fin" factor in? I do understand "fins" in the sense of x-wings and fishy patterns.

Please forgive my backwardness. I'm Joe Sudoku solver, not one of the programmers around here...but I'm trying!

Luke in Ca

[daj95376]

After staring at this PM for quite awhile, I finally understood. Now, let's hope that I can explain it.

Code: Select all

 *--------------------------------------------------------------------*
 | 56     26     462    | 1458   7      9      | 3      4612   1248   |
 | 1      9      4627   | 45+8   3      48     | 45+278 4627   248    |
 | 357    37     8      | 45+1   2      6      | 45+7   147    9      |
 |--------------------------------------------------------------------|
 | 4      2378   1      | 78     5      2378   | 289    239    6      |
 | 368    5      9      | 468    1468   12348  | 248    234    7      |
 | 3678   23678  2367   | 46789  4689   23478  | 1      5      2348   |
 |----------------------+----------------------+----------------------|
 | 2      178    5      | 3      1489   1478   | 6      1479   14     |
 | 36789  13678  367    | 2      14689  1478   | 479    13479  5      |
 | 3679   4      367    | 679    169    5      | 279    8      123    |
 *--------------------------------------------------------------------*


Code: Select all

(1) [r2c7]=4 => [r3c78]<>4 => [r3c4]=4

+-----------------------------------+
|  .  .  4  |  4  .  .  |  .  4  4  |
|  .  .  4  |  4  .  4  |  4  4  4  |
|  .  .  .  |  4  .  .  |  4  4  .  |
|-----------+-----------+-----------|
|  4  .  .  |  .  .  .  |  .  .  .  |
|  .  .  .  |  4  4  4  |  4  4  .  |
|  .  .  .  |  4  4  4  |  .  .  4  |
|-----------+-----------+-----------|
|  .  .  .  |  .  4  4  |  .  4  4  |
|  .  .  .  |  .  4  4  |  4  4  .  |
|  .  4  .  |  .  .  .  |  .  .  .  |
+-----------------------------------+


Code: Select all

(2) [r2c7]<>5 => [r2c4]=5 and [r3c7]=5

+-----------------------------------+
|  5  .  .  |  5  .  .  |  .  .  .  |
|  .  .  .  |  5  .  .  |  5  .  .  |
|  5  .  .  |  5  .  .  |  5  .  .  |
|-----------+-----------+-----------|
|  .  .  .  |  .  5  .  |  .  .  .  |
|  .  5  .  |  .  .  .  |  .  .  .  |
|  .  .  .  |  .  .  .  |  .  5  .  |
|-----------+-----------+-----------|
|  .  .  5  |  .  .  .  |  .  .  .  |
|  .  .  .  |  .  .  .  |  .  .  5  |
|  .  .  .  |  .  .  5  |  .  .  .  |
+-----------------------------------+


If (1) is true ... then (2) follows ... and [r23c47] would look like ...

Code: Select all

|  .  .  .  |  .  .  .  |  .  .  .  |
|  .  .  .  |  5  .  .  |  4  .  .  |
|  .  .  .  |  4  .  .  |  5  .  .  |


... which is a deadly pattern. Thus, we can conclude that [r2c7]<>4.

ronk: From your notation, it looks like you've spent a lot of time in the Eureka! forum lately.

[Steve K]

One way to look at any AUR is that is creates weak links, for example:
If ab is not given at.... then by AUR....groupC-groupD, one form of which is:
If 45 is not given at r23c47, then this is always true:
by AUR, (xwing5)r23c47-(xwing4)r23c47
On this particular puzzle, one could write the following AIC:sis means strong inference set
(5)r2c7=(5)r3c7-(5)r3c1=(rowxwing5)r23c47-(rowxwing4)r23c47=sis:[(4)r2c3589,r3c8] =>
r2c7<>4

[Mike Barker]

Looking at it another way. In the first example there are two strong links r2c7=5=r2c4 and r2c7=5=r3c7, but there is also a grouped strong link r3c78=4=r3c4. Thus if r2c7=4 then r2c4=5 and r3c7=5 and because of the weak link r2c7-4-r3c78, r3c4=4 creating the deadly pattern.

In the second example, there are the two strong links, r9c6=5=r4c6 and r9c6=5=r9c4, but the chain from r9c6 to r4c4 is missing so you can't make the elimination. In general if you have a UR+n (a UR with "n" nonbivalue cells) you need a combination of "n-1" strong or weak links (for example a bivalue) linking the target candidate to the other nonbivalue cells.

[eleven]

In the second example there is a simpler UR elimination, r6c4<>8.

[Luke]

Thanks to all who have tried to lift the fog. I'll study the responses over the weekend. I was lost, but now I'm loster....

Daj, this makes sense to me:

After staring at this PM for quite awhile, I finally understood. Now, let's hope that I can explain it.

Code: Select all

+-----------------------------------+
|  .  .  4  |  4  .  .  |  .  4  4  |
|  .  .  4  |  4  .  4  |  4  4  4  |
|  .  .  .  |  4  .  .  |  4  4  .  |
|-----------+-----------+-----------|
|  4  .  .  |  .  .  .  |  .  .  .  |
|  .  .  .  |  4  4  4  |  4  4  .  |
|  .  .  .  |  4  4  4  |  .  .  4  |
|-----------+-----------+-----------|
|  .  .  .  |  .  4  4  |  .  4  4  |
|  .  .  .  |  .  4  4  |  4  4  .  |
|  .  4  .  |  .  .  .  |  .  .  .  |
+-----------------------------------+


Code: Select all

(2) [r2c7]<>5 => [r2c4]=5 and [r3c7]=5

+-----------------------------------+
|  5  .  .  |  5  .  .  |  .  .  .  |
|  .  .  .  |  5  .  .  |  5  .  .  |
|  5  .  .  |  5  .  .  |  5  .  .  |
|-----------+-----------+-----------|
|  .  .  .  |  .  5  .  |  .  .  .  |
|  .  5  .  |  .  .  .  |  .  .  .  |
|  .  .  .  |  .  .  .  |  .  5  .  |
|-----------+-----------+-----------|
|  .  .  5  |  .  .  .  |  .  .  .  |
|  .  .  .  |  .  .  .  |  .  .  5  |
|  .  .  .  |  .  .  5  |  .  .  .  |
+-----------------------------------+


If (1) is true ... then (2) follows ... and [r23c47] would look like ...

Code: Select all

|  .  .  .  |  .  .  .  |  .  .  .  |
|  .  .  .  |  5  .  .  |  4  .  .  |
|  .  .  .  |  4  .  .  |  5  .  .  |


... which is a deadly pattern. Thus, we can conclude that [r2c7]<>4.

Now, this is proof, but it seems more like a trial and error approach rather than what Myth J was getting at. In other words, stick a four in there and see if a discrepancy arises, which, lo, it does.

Luke in Ca

[Luke]

I'm on a UR kick these days, so I'm poring over some of the older posts and digging up bodies.

here is a few more:

Unique Rectangle with 2 strong links:

Code: Select all

8       4       39      | 13      5       6       | 7       2       19
7       1       2       | 4       9       8       | 6       5       3
69      36      5       | 7       12      23      | 89      48      149
------------------------+-------------------------+------------------------
1349    5       8       | 1369    146     349     | 2       16      7
169     67      79      | 1569U-9 126     259U    | 4       3       8
1346    2       34      | 8       7       34      | 59      16      59
------------------------+-------------------------+------------------------
2       78      47      | 56      468     1       | 3       9       45
345     38      6       | 59U     48      459U    | 1       7       2
45      9       1       | 2       3       7       | 58      48      6


There are strongs links on <5> in the rectangle, another in blue on <9>. The elimination is in the box.



Is this elimination related to the red links, the blue, or both? Does this have something to do with this rule of Harvard's?

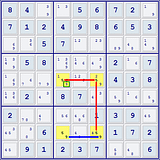
Havard 4-8-06
U meets xwing page 1

With a Unique Rectangle with the numbers [ab] that has two cells with
"extra candidates" ([xabx]), and these cells are on a diagonal from each other
and you have a strong link between any two of the cells in the Rectangle for candidate [a],
then you can eliminate candidate [a] from the cell with extra
candidates that is not part of the strong link.

As I understand it, this rule only applies to rectangles with bivalues in
diagonal squares and extras in the other two cells.

Thanks from Luke in Ca

[Luke]

I regards to the previous puzzle, there is a UR elimination I see quite often
right in the same rectangle being discussed:

Code: Select all


8       4       39      | 13      5       6       | 7       2       19
7       1       2       | 4       9       8       | 6       5       3
69      36      5       | 7       12      23      | 89      48      149
------------------------+-------------------------+------------------------
1349    5       8       | 1369    146     349     | 2       16      7
169     67      79      | 1569U   126     259U-9   | 4       3       8
1346    2       34      | 8       7       34      | 59      16      59
------------------------+-------------------------+------------------------
2       78      47      | 56      468     1       | 3       9       45
345     38      6       | 59U     48      459U    | 1       7       2
45      9       1       | 2       3       7       | 58      48      6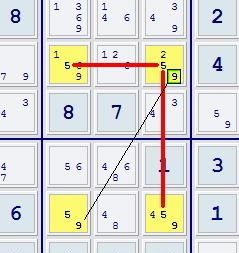

Whenever there's a "strong elbow" on the diagonal of a bivalue cell,
the base component not involved with the strong links can be eliminated
from the "elbow" cell.

In this puzzle, the strong links in red on <5> form the elbow on the diagonal
of the bivalue cell <59>. The uninvolved candidate <9> can never be in that cell,
so it gets elbowed out.

Luke in Ca

[Luke]

Thanks to all for helping shed light on my minor mysteries.....

ravel/myth jellies wrote:

Code: Select all

 *--------------------------------------------------------------------*
 | 56     26     462    | 1458   7      9      | 3      4612   1248   |
 | 1      9      4627   | 45+8   3      48     | 45+278 4627   248    |
 | 357    37     8      | 45+1   2      6      | 45+7   #147    9      |
 |--------------------------------------------------------------------|
 | 4      2378   1      | 78     5      2378   | 289    239    6      |
 | 368    5      9      | 468    1468   12348  | 248    234    7      |
 | 3678   23678  2367   | 46789  4689   23478  | 1      5      2348   |
 |----------------------+----------------------+----------------------|
 | 2      178    5      | 3      1489   1478   | 6      1479   14     |
 | 36789  13678  367    | 2      14689  1478   | 479    13479  5      |
 | 3679   4      367    | 679    169    5      | 279    8      123    |
 *--------------------------------------------------------------------*

...since its going crazy anyway: what about the elimination rep'nA found in the grid MJ showed above? You have 2 strong links for the 5 and a "finned" strong link for the 4 to eliminate 4 in r2c7.


Hmmm. It is pretty easy to show that when you have just a "strong elbow" in a generic UR(+4, though it doesn't have to be +4)...

Code: Select all


abX-----abZ
     a  /|
         |
     /  a|
         |
  /      |
abY     abW

...the 'b' candidate in the corner of the elbow is weakly linked to either the 'a' or 'b' candidates in the cell in the opposite corner. In other words, if either 'a' or 'b' are true in the abY-cell then b is false in the abZ-cell, and vice-versa. Any conjugate or strong link between the 'b' in the abY-cell and any grouping of 'b's sharing a house with the abZ-cell will thus kill the 'b' in the abZ-cell. This particular case is in the realm of an AUR, but it is quite obvious to see--probably as easy to spot as a type 3/UR assisted locked set deduction.

The part that intrigues me about this is,

"The 'b' candidate in the corner of the elbow is weakly linked to either the 'a' or 'b' candidates in the cell in the opposite corner."

This is helping me answer my original question, along with those who have pointed out the importance of the grouped strong link on <4> in row 3.

So, now.... ... What's to keep one from looking at this as a wee X-cycle, or "grouped discontinuous alternating nice loop?" Can one follow the diagonals in loops like these? Say, like 4[r2c7] - 4[r3c4] = 4[r3c78] - 4[r2c7], like this??




I've always just followed the straight and narrow, row and column. I don't know if we're allowed to color outside the lines....

Luke in Ca

[Mike Barker]

Havard's rule deals with a UR with two non-bivalued UR, but the same logic applies here. If r5c4=9, then the weak link to r8c4 forces r8c6=9 via the strong link r8c4=9=r8c6, but you also need the direct link, r5c4=5=r5c6, to force r5c6=5 to create the deadly pattern. Its also easy to show that r5c6<>9 because it directly links to both r5c4 (r5c4=5=r5c6) and r8c6 (r5c6=5=r8c6) via the strong links.

Code: Select all

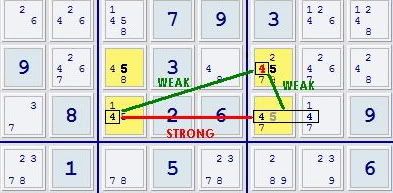
8       4       39      | 13      5       6       | 7       2       19
7       1       2       | 4       9       8       | 6       5       3
69      36      5       | 7       12      23      | 89      48      149
------------------------+-------------------------+------------------------
1349    5       8       | 1369    146     349     | 2       16      7
169     67      79      | 156-9*  126     25-9*   | 4       3       8
1346    2       34      | 8       7       34      | 59      16      59
------------------------+-------------------------+------------------------
2       78      47      | 56      468     1       | 3       9       45
345     38      6       | 59*     48      459*    | 1       7       2
45      9       1       | 2       3       7       | 58      48      6

These are examples of the many types of UR's described here and here.

4[r2c7] - 4[r3c4] = 4[r3c78] - 4[r2c7] is not valid since r2c7 doesn't see r3c4

[Myth Jellies]

Code: Select all

 *--------------------------------------------------------------------*
 | 56     26     462    | 1458   7      9      | 3      4612   1248   |
 | 1      9      4627   | 45+8   3      48     | 45+278 4627   248    |
 | 357    37     8      | 45+1   2      6      | 45+7   147    9      |
 |--------------------------------------------------------------------|
 | 4      2378   1      | 78     5      2378   | 289    239    6      |
 | 368    5      9      | 468    1468   12348  | 248    234    7      |
 | 3678   23678  2367   | 46789  4689   23478  | 1      5      2348   |
 |----------------------+----------------------+----------------------|
 | 2      178    5      | 3      1489   1478   | 6      1479   14     |
 | 36789  13678  367    | 2      14689  1478   | 479    13479  5      |
 | 3679   4      367    | 679    169    5      | 279    8      123    |
 *--------------------------------------------------------------------*

Often with AURs it is easier to find a deduction if you concentrate on where the base digits can escape the deadly pattern. If the elbow digit escapes the pattern, then the elbow must always be that digit. Hence if the 5's escape then the elbow (r2c7) must be 5. If the 4's in box 2 escape then it is easy to see that you have an r12/b12 box-box deduction that eliminates 4 in the elbow (or alternatively a grouped strong link for the 4's in row 3). Obviously if the 4's in box 3 escape then there is no 4 in the elbow as well. Since those are your only deadly pattern avoidance options, r2c7 <> 4.

Your outside the lines link works as well, Luke. I think your diagram shows very well what I was trying to say a long time ago.

The upshot is, whenever you spot a strong elbow, look for a potential UR that overlays it--it may lead to a reasonably quick elimination.

[Luke]

Thanks for all your help. I'm starting to catch on....

While searching for unique rectangles I’m finding that it’s worthwhile to pay attention to strong links even if there’s no AUR to be found. There often is an elimination within the rectangle by other means.

Here’s Ruud’s Nightmare from Saturday, 11/10/07:

Code: Select all

 *-----------*
 |..8|..5|..2|
 |..4|8..|...|
 |1..|3..|.8.|
 |---+---+---|
 |.9.|...|.37|
 |...|6.3|...|
 |84.|...|.6.|
 |---+---+---|
 |.3.|..6|..5|
 |...|..7|9..|
 |2..|4..|7..|
 *-----------*

 
 *-----------------------------------------------------------*
 | 369   67    8     | 179   4     5     | 136   79    2     |
 | 359   2567  4     | 8     267   129   | 356   579   19    |
 | 1     2567  569   | 3     2679  29    | 56    8     4     |
 |-------------------+-------------------+-------------------|
 | 56    9     256   | 125   8     4     | 125   3     7     |
 | 57    1     257   | 6     259   3     | 4     59    8     |
 | 8     4     3     | 2579  2579  129   | 25    6     19    |
 |-------------------+-------------------+-------------------|
 | 479   3     179   | 29    129   6     | 8     24    5     |
 | 456   8     156   | 25    1235  7     | 9     24    36    |
 | 2     56    69    | 4     359   8     | 7     1     36    |
 *-----------------------------------------------------------*


This rectangle has strong links on 2, 6, and 7. That’s a lot for one little rectangle! The trick seems to be to check these three values in the cells not involved with their strong links. For example, look at the 6 in r2c2. 6 is involved with a strong link within the rectangle, but not in this cell. If the 6 is in this cell you can tell at a glance there’ll be no home for 6 at all in box 2. As a discontinuity you could write 6[r2c2] -6[r2c5] =6[r3c5] -7[r3c5] =7[r3c2] -2[r3c2] =2[r2c2] -6[r2c2], so the 6 is history.

Same thing with the 2 in r2c5. 2 is involved with a strong link within the rectangle but not in this cell. All heck breaks loose if the 2 is in this cell, what with all the strong links. 2[r2c5] -6[r2c5] =6[r3c5] -7[r3c5] =7[r3c2] -2[r3c2] =2[r2c2] - 2[r2c5], and 2 is also gone.

.....................................................................................................................

Same puzzle, another rectangle with strong links on 1 and 2 and a grouped strong link on 5.





The 5 in r4c4 catches my attention because 5 is involved with a strong link but not in this cell. Sure enough, if 5 goes there, there’s no home for 5 at all in row 6 due to all the strong links. Of course, a discontinuous AIC proves it. (There may also be a AUR in this rectangle that I'm not catching.)

Luke in Ca