Impossible situations?
10 posts
• Page 1 of 1
Impossible situations?
Is it always possible to place a template if in each row, each column and each region we have two empty cells?
- Maq777
- Posts: 56
- Joined: 30 April 2016
Re: Impossible situations?
if from any resolution state you can't get to at least one full set of nine disjoint templates you don't have a valid puzzle
- P.O.
- Posts: 2071
- Joined: 07 June 2021
Re: Impossible situations?
Maq777 wrote:Is it always possible to place a template if in each row, each column and each region we have two empty cells?
in this case [which doesnt quite meet your criteria]... its not possible...
- Code: Select all
+---+---+---+
|...|...|..?|
|1..|2..|...|
|2..|1..|...|
+---+---+---+
|...|...|...|
|...|...|21.|
|...|...|...|
+---+---+---+
|...|...|...|
|...|...|...|
|...|...|12.|
+---+---+---+
- coloin
- Posts: 2646
- Joined: 05 May 2005
- Location: Devon
Re: Impossible situations?
Here is the example I found
- Code: Select all
+---+---+---+
|?**|***|**?|
|*?*|?**|***|
|***|*?*|?**|
+---+---+---+
|**?|*?*|***|
|?**|**?|***|
|***|***|??*|
+---+---+---+
|*?*|?**|***|
|***|***|*??|
|**?|**?|***|
+---+---+---+
+---+---+---+
|1**|***|**9|
|*9*|1**|***|
|***|*9*|1**|
+---+---+---+
|**1|*?*|***|
|9**|**?|***|
|***|***|91*|
+---+---+---+
|*1*|9**|***|
|***|***|*91|
|**9|**1|***|
+---+---+---+
- Maq777
- Posts: 56
- Joined: 30 April 2016
Re: Impossible situations?
all valid combinations of seven templates can't be completed to valid nine templates sudokus
- Code: Select all
58.72643.462.3857..374.5628.23.6784584635.7.275.2843.66748..2533.5672.842.8543.67 invalid
58.72643.462.3857.73.4.5628.23.6784584635.7.2.572843.66748..2533.5672.842.8543.67 4 solutions
76.54832..532.76484826.3.57627.34.8583..752645.48267.3..835247627648.53.34576.8.2 invalid
46.5.8327.532.7648782643.5.627834..583..752645.4.26783..835247627648.53.34576.8.2 4 solutions
4.35.872656.372.48728.643.5875.23.64..284653734675.28.2546378...3.4856726872..453 invalid
32.76.548648.352.7.574826.3..562783426384..757845.3.26876..435253.27846.4.235678. 2 solutions
- P.O.
- Posts: 2071
- Joined: 07 June 2021
Re: Impossible situations?
Maq777 wrote:Here is the example I found
- Code: Select all
+---+---+---+
|?**|***|**?|
|*?*|?**|***|
|***|*?*|?**|
+---+---+---+
|**?|*?*|***|
|?**|**?|***|
|***|***|??*|
+---+---+---+
|*?*|?**|***|
|***|***|*??|
|**?|**?|***|
+---+---+---+
+---+---+---+
|1**|***|**9|
|*9*|1**|***|
|***|*9*|1**|
+---+---+---+
|**1|*?*|***|
|9**|**?|***|
|***|***|91*|
+---+---+---+
|*1*|9**|***|
|***|***|*91|
|**9|**1|***|
+---+---+---+
r5c5=1, r4c6=9, the question marks are wrongly placed ??
-
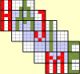
Hajime - Posts: 1405
- Joined: 20 April 2018
- Location: Fryslân
Re: Impossible situations?
In my case, it occurred when trying to create a sudoku using these seven templates, but I ended up in an impossible situation to continue.
.2345678.8.4.273565678.3.2428.3.4567.3567.842476285..33.8.426756527384..74.56.238
.2345678.8.4.273565678.3.2428.3.4567.3567.842476285..33.8.426756527384..74.56.238
- Maq777
- Posts: 56
- Joined: 30 April 2016
Re: Impossible situations?
suppose you build the puzzle one template at a time in ascending order the process would be as follows:
number of templates to choose from / your choice / the resulting puzzle
the puzzle that results from the choice of the template for 7 is found invalid as soon as it is initialized:
for each of the three values 1,8 and 9 the same 11 templates are available, but none of the cells (1 13 25 53) are in them so the candidate for each of the three values must be eliminated from them leaving four empty cells
also once the choice for value 8 is made from one of the 11 available templates, from the 18 remaining cells 48620 combinations of 9 cells can be made none of which has its 9 cells pairwise disconnected
number of templates to choose from / your choice / the resulting puzzle
Hidden Text: Show
the puzzle that results from the choice of the template for 7 is found invalid as soon as it is initialized:
for each of the three values 1,8 and 9 the same 11 templates are available, but none of the cells (1 13 25 53) are in them so the candidate for each of the three values must be eliminated from them leaving four empty cells
also once the choice for value 8 is made from one of the 11 available templates, from the 18 remaining cells 48620 combinations of 9 cells can be made none of which has its 9 cells pairwise disconnected
Hidden Text: Show
- P.O.
- Posts: 2071
- Joined: 07 June 2021
Re: Impossible situations?
Sure thing! You just need to make sure you've got all your numbers in the right spots--that'll leave ya with two empties per row, column and region every single time.
- sudokuvalleyvenusrye
- Posts: 6
- Joined: 12 June 2023
Re: Impossible situations?
Exactly that seemed strange to me from the situation that I showed, I have two empty cells for each row, for each column and for each region, however the puzzle cannot be completed. I didn't know that could happen.
- Maq777
- Posts: 56
- Joined: 30 April 2016
10 posts
• Page 1 of 1

